Stardate 20031030.0956

(Captain's log): Simon and Alvin are proud owners of brand new space ships, and decide to take them up to visit their friend Theodore at his space station in low earth orbit. Theodore is glad to see them, and soon they're singing songs together and discussing old times.

Alvin, always the troublemaker, starts insisting that his space ship is faster than Simon's. Simon points out that they're exactly the same model and equipped in exactly the same way, and should perform identically, but Alvin will have none of it, and eventually challenges Simon to a race. Simon and Alvin will start with their ships side by side next to Theodore's space station, and each of them will fire their engines for exactly 60 seconds. The first one to reach a point 10000 kilometers in front of Theodore's space station will be declared the winner. Theodore will judge the event, using the radar in his station.

Simon is a straightforward dude, and he faces his ship towards the goal and fires his engines for 60 seconds. Alvin, always a bit perverse, swings his ship around, faces directly away from the end point, and fires his engines for 60 seconds. Who wins the race?

What Theodore will observe is rather strange:

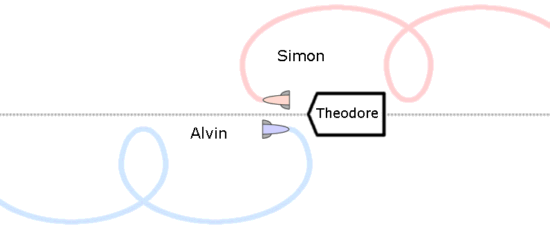
Which seems to make no sense. Why should they follow such strange paths, and why does Alvin win the race given that he seems to accelerate in the wrong direction?

There are a couple of major issues here. The first one is that I'm presenting the situation as Theodore sees it, which is to say that I'm using Theodore's space station as the frame of reference. We're dealing with the part of physics known as mechanics, which is the part of physics within which Galileo and Newton and Einstein did most of their major work. Mechanics more or less studies motion, acceleration, momentum and certain manifestations of energy. You have to choose a frame of reference, which is an arbitrary point and orientation which is defined as being coordinates (0,0,0) with a velocity of zero. All positions are given as offsets from the frame of reference, and all velocities are given as movement relative to the frame of reference.

But not all frames of reference are created equally. There are two kinds: inertial frames of reference and accelerating frames of reference.

Einstein's Special Theory of Relativity began with the problem of explaining why the value known to physicists as C (the speed of light in a vacuum) seems to be the same when measured within any inertial frame of reference. And it does explain it, but in doing so it revealed a lot of other things about the universe.

What Special Relativity tells us is that inertial frames of reference are mathematical conveniences which have no true existence. There's no actual zero-point in the universe which is "not moving" against which we can measure absolute velocities or absolute positions. All inertial frames of reference are equally valid; none is better than any other.

Accelerating frames of reference (which includes those which rotate) are not as kind to observers of physical phenomena. Most of what we think of as the classic laws of Mechanics don't apply to measurements taken within an accelerating frame of reference. For instance, neither kinetic energy nor momentum will necessarily be conserved. C isn't necessarily a constant; it can be measured as greater or less than the published number. Objects can seem to exceed the published speed of C. Force may not correlate to acceleration, and velocity can change without either force or acceleration. The problem is that accelerating frames of reference aren't really valid. It isn't possible to make meaningful observations and calculations based on one without correcting everything to an inertial frame of reference.

However, some accelerating frames of reference can be close enough to being inertial so that some kinds of measurements will be very close to valid. But other measurements in that same frame of reference can be completely useless. It's a trap you have to watch out for.

One of the two big reasons why the result seems strange is that I described the race from Theodore's point of view, using Theodore's space station as the frame of reference, and it is both accelerating and rotating. The apparent behavior is inexplicable because the frame of reference is invalid. I drew Theodore's motion as a straight line, but he's actually in orbit.

The other reason for this strange behavior is that orbital mechanics are counterintuitive: a force in your orbital direction adds energy to your orbit, which makes it slower.

All orbits are ellipses, with the gravitational center of the primary at one focus of the ellipse. (A circle is a special case of an ellipse, for purposes of orbital dynamics, and is treated as an ellipse where both focuses are at the same point.)

Here's what really happened in the Great Chipmunk Spaceship Race, using a valid inertial frame of reference:

Theodore's space station is more or less in a circular orbit, which is also the orbit that Alvin and Simon were in before the race began. When Simon fired his engines, he put himself into a elliptical orbit whose perigee (the low point in the orbit) was the same altitude as Theodore's, but whose apogee (the high point) was much higher. When Simon is at th